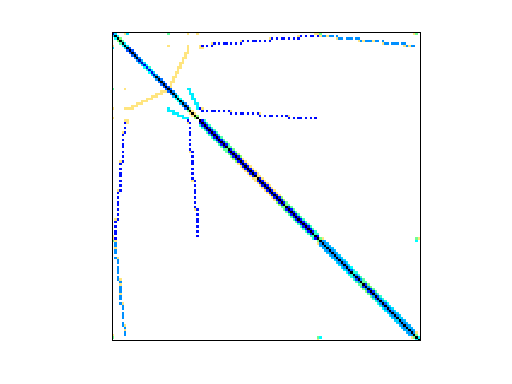
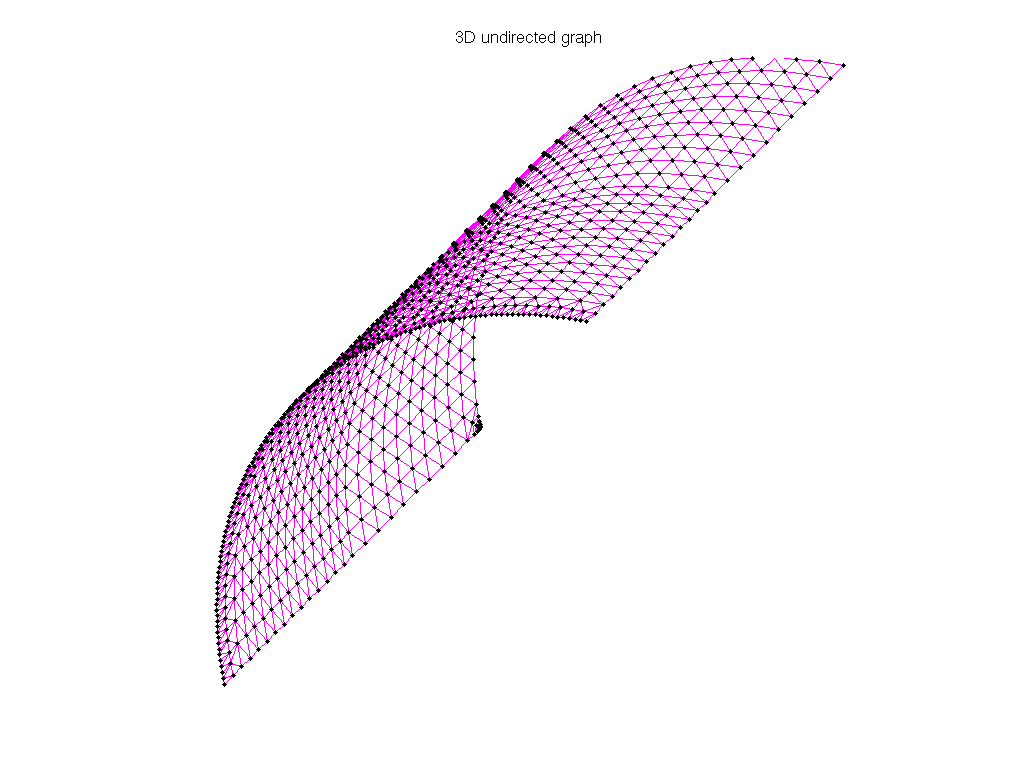
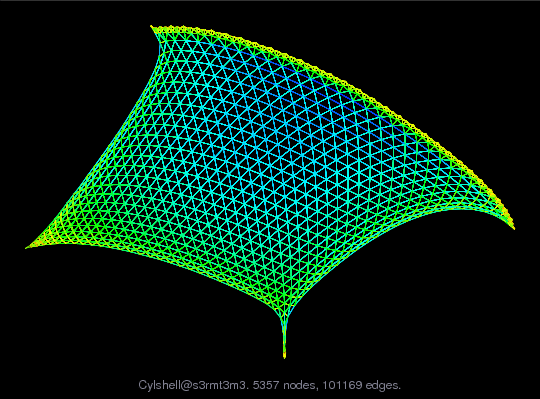
Cylshell/s3rmt3m3
FEM, cylindrical shell, graded tri. mesh w/ 1666 triangles. , R/t=1000
| Name |
s3rmt3m3 |
| Group |
Cylshell |
| Matrix ID |
1611 |
|
Num Rows
|
5,357 |
|
Num Cols
|
5,357 |
|
Nonzeros
|
207,123 |
|
Pattern Entries
|
207,695 |
|
Kind
|
Structural Problem |
|
Symmetric
|
Yes |
|
Date
|
1997 |
|
Author
|
R. Kouhia |
|
Editor
|
R. Boisvert, R. Pozo, K. Remington, B. Miller, R. Lipman, R. Barrett, J. Dongarra |
| Structural Rank |
5,357 |
| Structural Rank Full |
true |
|
Num Dmperm Blocks
|
1 |
|
Strongly Connect Components
|
1 |
|
Num Explicit Zeros
|
572 |
|
Pattern Symmetry
|
100% |
|
Numeric Symmetry
|
100% |
|
Cholesky Candidate
|
yes |
|
Positive Definite
|
yes |
|
Type
|
real |
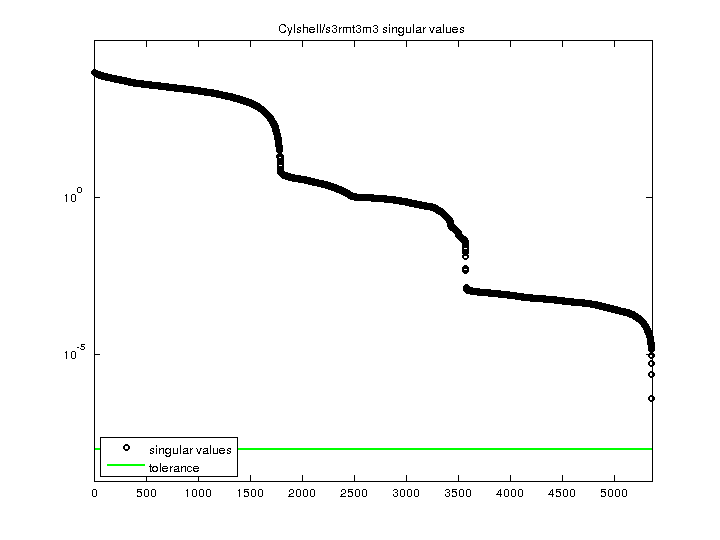
| SVD Statistics |
| Matrix Norm |
9.598608e+03 |
| Minimum Singular Value |
3.998353e-07 |
| Condition Number |
2.400640e+10
|
| Rank |
5,357 |
| sprank(A)-rank(A) |
0 |
| Null Space Dimension |
0 |
| Full Numerical Rank? |
yes |
| Download Singular Values |
MATLAB
|
| Download |
MATLAB
Rutherford Boeing
Matrix Market
|
| Notes |
%
%FILE s3rmt3m3.mtx
%TITLE Cyl shell R/t=1000 grad trian mesh 1666 stab MITC3 elem with drill rot
%KEY s3rmt3m3
%
%
%CONTRIBUTOR Reijo Kouhia (reijo.kouhia@hut.fi)
%
%BEGIN DESCRIPTION
% Matrix from a static analysis of a cylindrical shell
% Radius to thickness ratio R/t = 1000
% Length to radius ratio R/L = 1
% One octant discretized with graded triangular mesh (1666 elements)
% element:
% facet-type shell element where the bending part is formulated
% using the stabilized MITC theory (stabilization paramater 0.4)
% the membrane part includes drilling rotations using
% the Hughes-Brezzi formulation with (regularizing parameter = G/1000,
% where G is the shear modulus)
% full 3-point integration
% --------------------------------------------------------------------------
% Note:
% The sparsity pattern of the matrix is determined from the element
% connectivity data assuming that the element matrix is full.
% Since this case the material model is linear isotropically elastic
% there exist some zeros.
% Since the removal of those zero elements is trivial
% but the reconstruction of the current sparsity
% pattern is impossible from the sparsified structure without any further
% knowledge of the element connectivity, the zeros are retained in this file.
% ---------------------------------------------------------------------------
%END DESCRIPTION
%
%
|